Preface ...................................................... xiii
Jeffery McNeal and Mircea Mustaţă
Introduction .................................................... 1
Bo Berndtsson
An Introduction to Things ....................................... 7
Introduction .................................................... 9
Lecture 1 The one-dimensional case ........................... 11
1.1 The 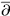 -equation in one variable ............................ 11
1.2 An alternative proof of the basic identity ............... 14
1.3 An application: Inequalities of Brunn-Minkowski type ...... 14
1.4 Regularity - a disclaimer ................................. 16
Lecture 2 Functional analytic interlude ....................... 19
2.1 Dual formulation of the -equation in one variable ............................ 11
1.2 An alternative proof of the basic identity ............... 14
1.3 An application: Inequalities of Brunn-Minkowski type ...... 14
1.4 Regularity - a disclaimer ................................. 16
Lecture 2 Functional analytic interlude ....................... 19
2.1 Dual formulation of the 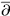 -problem ......................... 19
Lecture 3 The -problem ......................... 19
Lecture 3 The 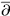 -equation on a complex manifold ................ 25
3.1 Metrics ................................................... 25
3.2 Norms of forms ............................................ 27
3.3 Line bundles .............................................. 29
3.4 Calculation of the adjoint and the basic identity ......... 33
3.5 The main existence theorem and L2-estimate for compact
manifolds ................................................. 35
3.6 Complete Kähler manifolds ................................. 37
Lecture 4 The Bergman kernel .................................. 43
4.1 Generalities .............................................. 43
4.2 Bergman kernels associated to complex line bundles ........ 46
Lecture 5 Singular metrics and the Kawamata-Viehweg
vanishing theorem ................................... 51
5.1 The Demailly-Nadel vanishing theorem ...................... 51
5.2 The Kodaira embedding theorem ............................. 54
5.3 The Kawamata-Viehweg vanishing theorem .................... 55
Lecture 6 Adjunction and extension from divisors .............. 59
6.1 Adjunction and the currents defined by divisors ........... 59
6.2 The Ohsawa-Takegoshi extension theorem .................... 62
Lecture 7 Deformational invariance of plurigenera ............. 71
7.1 Extension of pluricanonical forms ......................... 71
Bibliography .............................................. 75
John P. D'Angelo
Real and Complex Geometry meet the Cauchy-Riemann Equations .... 77
Preface ........................................................ 79
Lecture 1 Background material ................................. 81
1 Complex linear algebra ...................................... 81
2 Differential forms .......................................... 82
3 Solving the Cauchy-Riemann equations ........................ 84
Lecture 2 Complex varieties in real hypersurfaces ............. 87
1 Degenerate critical points of smooth functions .............. 87
2 Hermitian symmetry and polarization ......................... 89
3 Holomorphic decomposition ................................... 90
4 Real analytic hypersurfaces and subvarieties ................ 98
5 Complex varieties, local algebra, and multiplicities ........ 99
Lecture 3 Pseudoconvexity, the Levi form, and points of
finite type ........................................ 105
1 Euclidean convexity ........................................ 105
2 The Levi form .............................................. 107
3 Higher order commutators ................................... 111
4 Points of finite type ...................................... 113
5 Commutative algebra ........................................ 116
6 A return to finite type .................................... 121
7 The set of finite type points is open ...................... 126
Lecture 4 Kohn's algorithm for subelliptic multipliers ....... 129
1 Introduction ............................................... 129
2 Subelliptic estimates ...................................... 130
3 Kohn's algorithm ........................................... 133
4 Kohn's algorithm for holomorphic and formal germs .......... 134
5 Failure of effectiveness for Kohn's algorithm .............. 139
6 Triangular systems ......................................... 140
7 Additional remarks ......................................... 144
Lecture 5 Connections with partial differential equations .... 147
1 Finite type conditions ..................................... 147
2 Local regularity for -equation on a complex manifold ................ 25
3.1 Metrics ................................................... 25
3.2 Norms of forms ............................................ 27
3.3 Line bundles .............................................. 29
3.4 Calculation of the adjoint and the basic identity ......... 33
3.5 The main existence theorem and L2-estimate for compact
manifolds ................................................. 35
3.6 Complete Kähler manifolds ................................. 37
Lecture 4 The Bergman kernel .................................. 43
4.1 Generalities .............................................. 43
4.2 Bergman kernels associated to complex line bundles ........ 46
Lecture 5 Singular metrics and the Kawamata-Viehweg
vanishing theorem ................................... 51
5.1 The Demailly-Nadel vanishing theorem ...................... 51
5.2 The Kodaira embedding theorem ............................. 54
5.3 The Kawamata-Viehweg vanishing theorem .................... 55
Lecture 6 Adjunction and extension from divisors .............. 59
6.1 Adjunction and the currents defined by divisors ........... 59
6.2 The Ohsawa-Takegoshi extension theorem .................... 62
Lecture 7 Deformational invariance of plurigenera ............. 71
7.1 Extension of pluricanonical forms ......................... 71
Bibliography .............................................. 75
John P. D'Angelo
Real and Complex Geometry meet the Cauchy-Riemann Equations .... 77
Preface ........................................................ 79
Lecture 1 Background material ................................. 81
1 Complex linear algebra ...................................... 81
2 Differential forms .......................................... 82
3 Solving the Cauchy-Riemann equations ........................ 84
Lecture 2 Complex varieties in real hypersurfaces ............. 87
1 Degenerate critical points of smooth functions .............. 87
2 Hermitian symmetry and polarization ......................... 89
3 Holomorphic decomposition ................................... 90
4 Real analytic hypersurfaces and subvarieties ................ 98
5 Complex varieties, local algebra, and multiplicities ........ 99
Lecture 3 Pseudoconvexity, the Levi form, and points of
finite type ........................................ 105
1 Euclidean convexity ........................................ 105
2 The Levi form .............................................. 107
3 Higher order commutators ................................... 111
4 Points of finite type ...................................... 113
5 Commutative algebra ........................................ 116
6 A return to finite type .................................... 121
7 The set of finite type points is open ...................... 126
Lecture 4 Kohn's algorithm for subelliptic multipliers ....... 129
1 Introduction ............................................... 129
2 Subelliptic estimates ...................................... 130
3 Kohn's algorithm ........................................... 133
4 Kohn's algorithm for holomorphic and formal germs .......... 134
5 Failure of effectiveness for Kohn's algorithm .............. 139
6 Triangular systems ......................................... 140
7 Additional remarks ......................................... 144
Lecture 5 Connections with partial differential equations .... 147
1 Finite type conditions ..................................... 147
2 Local regularity for 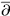 ..................................... 149
3 Hypoellipticity, global regularity, and compactness ........ 150
4 An introduction to L2-estimates ............................ 152
Lecture 6 Positivity conditions .............................. 157
1 Introduction ............................................... 157
2 The classes ..................................... 149
3 Hypoellipticity, global regularity, and compactness ........ 150
4 An introduction to L2-estimates ............................ 152
Lecture 6 Positivity conditions .............................. 157
1 Introduction ............................................... 157
2 The classes 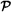 k ............................................. 158
3 Intermediate conditions .................................... 159
4 The global Cauchy-Schwarz inequality ....................... 161
5 A complicated example ...................................... 164
6 Stabilization in the bihomogeneous polynomial case ......... 166
7 Squared norms and proper mappings between balls ............ 171
8 Holomorphic line bundles ................................... 173
Lecture 7 Some open problems ................................. 175
Bibliography ............................................... 177
Dror Varolin
Three Variations on a Theme in Complex Analytic Geometry ...... 183
Lecture 8. Basic notions in complex geometry ................ 189
1 Complex manifolds .......................................... 189
2 Connections ................................................ 199
3 Curvature .................................................. 207
4 Holomorphic line bundles ................................... 210
Lecture 1 The Hörmander theorem .............................. 217
1 Functional analysis ........................................ 218
2 The Bochner-Kodaira identity ............................... 219
3 Manifolds with boundary .................................... 226
4 Density of smooth forms in the graph norm .................. 229
5 Hörmander's theorem ........................................ 234
6 Singular Hermitian metrics for line bundles ................ 236
7 Application: Kodaira embedding theorem ..................... 239
8 Multiplier ideal sheaves and Nadel's Theorems .............. 242
9 Exercises .................................................. 248
Lecture 2 The L2 extension theorem ........................... 251
1 L2 extension ............................................... 251
2 The deformation invariance of plurigenera .................. 259
3 Pluricanonical extension on projective manifolds ........... 265
4 Exercises .................................................. 275
Lecture 3 The Skoda division theorem ......................... 277
1 Statement of the division theorem .......................... 277
2 Proof of the division theorem .............................. 278
3 Global generation of multiplier ideal sheaves .............. 286
4 Exercises .................................................. 290
Bibliography ............................................... 293
Jean-Pierre Demailly
Structure Theorems for Projective and Kähler Varieties ........ 295
0 Introduction ............................................... 297
1 Numerically effective and pseudo-effective (1,1) classes ... 298
1.A. Pseudo-effective line bundles and metrics with
minimal singularities ................................. 298
1.B. Nef line bundles ...................................... 300
1.C. Description of the positive cones ..................... 302
1.D. The Kawamata-Viehweg vanishing theorem ................ 306
1.E. A uniform global generation property due to
Y.T. Siu .............................................. 308
1.F. Hard Lefschetz theorem with multiplier ideal
sheaves ............................................... 309
2 Holomorphic Morse inequalities ............................. 310
3 Approximation of closed positive (l,l)-currents by
divisors ................................................... 312
3.A. Local approximation theorem through Bergman kernels ... 312
3.B. Global approximation of closed (l,l)-currents on
a compact complex manifold ............................ 314
3.C. Global approximation by divisors ...................... 320
3.D. Singularity exponents and log canonical thresholds .... 326
4 Subadditivity of multiplier ideals and Fujita's
approximate Zariski decomposition theorem .................. 329
5 Numerical characterization of the Kähler cone .............. 334
5.A. Positive classes in intermediate (p,p) bidegrees ...... 334
5.B. Numerically positive classes of type (1,1) ............ 335
5.C. Deformations of compact Kähler manifolds .............. 341
6 Structure of the pseudo-effective cone and mobile
intersection theory ........................................ 343
6.A. Classes of mobile curves and of mobile (n — 1,
n — l)-currents ....................................... 343
6.B. Zariski decomposition and mobile intersections ........ 346
6.C. The orthogonality estimate ............................ 352
6.D. Dual of the pseudo-effective cone ..................... 354
7 Super-canonical metrics and abundance ...................... 357
8.A. Construction of super-canonical metrics ............... 357
7.B. Invariance of plurigenera and positivity of
curvature of super-canonical metrics .................. 363
7.C. Tsuji's strategy for studying abundance ............... 364
8 Siu's analytic approach and Păun's non vanishing theorem ... 365
Bibliography ............................................... 367
Mihai Păun
Lecture Notes on Rational Polytopes and Finite Generation ..... 371
0 Introduction ............................................... 373
1 Basic definitions and notations ............................ 374
2 Proof of (i) ............................................... 376
2.1 The case nd({Kx + Yτ0 + A}) = 0 ....................... 377
2.2 The "x method" for sequences .......................... 378
2.3 The induced polytope and its properties ............... 382
3 Proof of (ii) .............................................. 390
3.1 The first step ........................................ 393
3.2 Iteration scheme ...................................... 399
3.1 References ............................................ 402
Mircea Mustaţă
Introduction to Resolution of Singularities ................... 405
Lecture 1 Resolutions and principalizations .................. 409
1.1 The main theorems ........................................ 409
1.2 Strengthenings of Theorem 1.3 ............................ 410
1.3 Historical comments ...................................... 414
Lecture 2 Marked ideals ...................................... 415
2.1 Marked ideals ............................................ 415
2.2 Derived ideals ........................................... 419
Lecture 3 Hypersurfaces of maximal contact and coefficient
ideals ............................................. 423
3.1 Hypersurfaces of maximal contact ......................... 423
3.2 The coefficient ideal .................................... 424
Lecture 4 Homogenized ideals ................................. 431
4.1 Basics of homogenized ideals ............................. 431
4.2 Comparing hypersurfaces of maximal contact: formal
equivalence .............................................. 433
4.3 Comparing hypersurfaces of maximal contact: etale
equivalence .............................................. 434
Lecture 5 Proof of principalization .......................... 437
5.1 The statements ........................................... 437
5.2 Part I: the maximal order case ........................... 438
5.3 Part II: the general case ................................ 442
5.4 Proof of principalization ................................ 445
Bibliography ............................................. 449
Robert Lazarsfeld
A Short Course on Multiplier Ideals ........................... 451
Introduction .................................................. 453
Lecture 1 Construction and examples of multiplier ideals ..... 455
Definition of multiplier ideals ............................... 455
Monomial ideals ............................................... 459
Invariants defined by multiplier ideals ....................... 460
Lecture 2 Vanishing theorems for multiplier ideals ........... 463
The Kawamata-Viehweg-Nadel vanishing theorem .................. 463
Singularities of plane curves and projective hypersurfaces .... 465
Singularities of theta divisors ............................... 467
Uniform global generation ..................................... 468
Lecture 3 Local properties of multiplier ideals .............. 471
Adjoint ideals and the restriction theorem .................... 471
The subadditivity theorem ..................................... 474
Skoda's theorem ............................................... 475
Lecture 4 Asymptotic constructions ........................... 479
Asymptotic multiplier ideals .................................. 479
Variants ...................................................... 482
Étale multiplicativity of plurigenera ......................... 484
A comparison theorem for symbolic powers ...................... 485
Lecture 5 Extension theorems and deformation invariance of
plurigenera ........................................ 487
Bibliography .................................................. 493
János Kollár
Exercises in the Birational Geometry of Algebraic Varieties ... 495
1 Birational classification of algebraic surfaces ............ 497
2 Naive minimal models ....................................... 498
3 The cone of curves ......................................... 503
4 Singularities .............................................. 508
5 Flips ...................................................... 513
6 Minimal models ............................................. 518
Bibliography ............................................... 523
Christopher D. Hacon
Higher Dimensional Minimal Model Program for Varieties of
Log General Type .............................................. 525
Introduction .................................................. 527
Lecture 1 Pl-flips ........................................... 531
Lecture 2 Multiplier ideal sheaves ........................... 535
Asymptotic multiplier ideal sheaves ........................... 538
Extending pluricanonical forms ................................ 540
Lecture 3 Finite generation of the restricted algebra ........ 545
Rationality of the restricted algebra ......................... 545
Proof of (1.10) ............................................... 546
Lecture 4 The minimal model program with scaling ............. 547
Solutions to the exercises .................................... 551
Bibliography .................................................. 555
Alessio Corti, Paul Hacking, János Kollár, Robert
Lazarsfeld, and Mircea Mustaţă
Lectures on Flips and Minimal Models .......................... 557
Lecture 1 Extension theorems ................................. 561
1.1 Multiplier and adjoint ideals ............................ 561
1.2 Proof of the Main Lemma .................................. 563
Lecture 2 Existence of flips I ............................... 565
2.1 The setup ................................................ 565
2.2 Adjoint algebras ......................................... 566
2.3 The Hacon-McKernan extension theorem ..................... 567
2.4 The restricted algebra as an adjoint algebra ............. 567
Lecture 3 Existence of flips II .............................. 571
Lecture 4 Notes on Birkar-Cascini-Hacon-McKernan ............. 575
4.1 Comparison of 3 MMP's .................................... 576
4.2 MMP with scaling ......................................... 578
4.3 MMP with scaling near |Δ| ................................ 578
4.4 Bending it like BCHM ..................................... 579
4.5 Finiteness of models ..................................... 581
Bibliography ............................................. 583 k ............................................. 158
3 Intermediate conditions .................................... 159
4 The global Cauchy-Schwarz inequality ....................... 161
5 A complicated example ...................................... 164
6 Stabilization in the bihomogeneous polynomial case ......... 166
7 Squared norms and proper mappings between balls ............ 171
8 Holomorphic line bundles ................................... 173
Lecture 7 Some open problems ................................. 175
Bibliography ............................................... 177
Dror Varolin
Three Variations on a Theme in Complex Analytic Geometry ...... 183
Lecture 8. Basic notions in complex geometry ................ 189
1 Complex manifolds .......................................... 189
2 Connections ................................................ 199
3 Curvature .................................................. 207
4 Holomorphic line bundles ................................... 210
Lecture 1 The Hörmander theorem .............................. 217
1 Functional analysis ........................................ 218
2 The Bochner-Kodaira identity ............................... 219
3 Manifolds with boundary .................................... 226
4 Density of smooth forms in the graph norm .................. 229
5 Hörmander's theorem ........................................ 234
6 Singular Hermitian metrics for line bundles ................ 236
7 Application: Kodaira embedding theorem ..................... 239
8 Multiplier ideal sheaves and Nadel's Theorems .............. 242
9 Exercises .................................................. 248
Lecture 2 The L2 extension theorem ........................... 251
1 L2 extension ............................................... 251
2 The deformation invariance of plurigenera .................. 259
3 Pluricanonical extension on projective manifolds ........... 265
4 Exercises .................................................. 275
Lecture 3 The Skoda division theorem ......................... 277
1 Statement of the division theorem .......................... 277
2 Proof of the division theorem .............................. 278
3 Global generation of multiplier ideal sheaves .............. 286
4 Exercises .................................................. 290
Bibliography ............................................... 293
Jean-Pierre Demailly
Structure Theorems for Projective and Kähler Varieties ........ 295
0 Introduction ............................................... 297
1 Numerically effective and pseudo-effective (1,1) classes ... 298
1.A. Pseudo-effective line bundles and metrics with
minimal singularities ................................. 298
1.B. Nef line bundles ...................................... 300
1.C. Description of the positive cones ..................... 302
1.D. The Kawamata-Viehweg vanishing theorem ................ 306
1.E. A uniform global generation property due to
Y.T. Siu .............................................. 308
1.F. Hard Lefschetz theorem with multiplier ideal
sheaves ............................................... 309
2 Holomorphic Morse inequalities ............................. 310
3 Approximation of closed positive (l,l)-currents by
divisors ................................................... 312
3.A. Local approximation theorem through Bergman kernels ... 312
3.B. Global approximation of closed (l,l)-currents on
a compact complex manifold ............................ 314
3.C. Global approximation by divisors ...................... 320
3.D. Singularity exponents and log canonical thresholds .... 326
4 Subadditivity of multiplier ideals and Fujita's
approximate Zariski decomposition theorem .................. 329
5 Numerical characterization of the Kähler cone .............. 334
5.A. Positive classes in intermediate (p,p) bidegrees ...... 334
5.B. Numerically positive classes of type (1,1) ............ 335
5.C. Deformations of compact Kähler manifolds .............. 341
6 Structure of the pseudo-effective cone and mobile
intersection theory ........................................ 343
6.A. Classes of mobile curves and of mobile (n — 1,
n — l)-currents ....................................... 343
6.B. Zariski decomposition and mobile intersections ........ 346
6.C. The orthogonality estimate ............................ 352
6.D. Dual of the pseudo-effective cone ..................... 354
7 Super-canonical metrics and abundance ...................... 357
8.A. Construction of super-canonical metrics ............... 357
7.B. Invariance of plurigenera and positivity of
curvature of super-canonical metrics .................. 363
7.C. Tsuji's strategy for studying abundance ............... 364
8 Siu's analytic approach and Păun's non vanishing theorem ... 365
Bibliography ............................................... 367
Mihai Păun
Lecture Notes on Rational Polytopes and Finite Generation ..... 371
0 Introduction ............................................... 373
1 Basic definitions and notations ............................ 374
2 Proof of (i) ............................................... 376
2.1 The case nd({Kx + Yτ0 + A}) = 0 ....................... 377
2.2 The "x method" for sequences .......................... 378
2.3 The induced polytope and its properties ............... 382
3 Proof of (ii) .............................................. 390
3.1 The first step ........................................ 393
3.2 Iteration scheme ...................................... 399
3.1 References ............................................ 402
Mircea Mustaţă
Introduction to Resolution of Singularities ................... 405
Lecture 1 Resolutions and principalizations .................. 409
1.1 The main theorems ........................................ 409
1.2 Strengthenings of Theorem 1.3 ............................ 410
1.3 Historical comments ...................................... 414
Lecture 2 Marked ideals ...................................... 415
2.1 Marked ideals ............................................ 415
2.2 Derived ideals ........................................... 419
Lecture 3 Hypersurfaces of maximal contact and coefficient
ideals ............................................. 423
3.1 Hypersurfaces of maximal contact ......................... 423
3.2 The coefficient ideal .................................... 424
Lecture 4 Homogenized ideals ................................. 431
4.1 Basics of homogenized ideals ............................. 431
4.2 Comparing hypersurfaces of maximal contact: formal
equivalence .............................................. 433
4.3 Comparing hypersurfaces of maximal contact: etale
equivalence .............................................. 434
Lecture 5 Proof of principalization .......................... 437
5.1 The statements ........................................... 437
5.2 Part I: the maximal order case ........................... 438
5.3 Part II: the general case ................................ 442
5.4 Proof of principalization ................................ 445
Bibliography ............................................. 449
Robert Lazarsfeld
A Short Course on Multiplier Ideals ........................... 451
Introduction .................................................. 453
Lecture 1 Construction and examples of multiplier ideals ..... 455
Definition of multiplier ideals ............................... 455
Monomial ideals ............................................... 459
Invariants defined by multiplier ideals ....................... 460
Lecture 2 Vanishing theorems for multiplier ideals ........... 463
The Kawamata-Viehweg-Nadel vanishing theorem .................. 463
Singularities of plane curves and projective hypersurfaces .... 465
Singularities of theta divisors ............................... 467
Uniform global generation ..................................... 468
Lecture 3 Local properties of multiplier ideals .............. 471
Adjoint ideals and the restriction theorem .................... 471
The subadditivity theorem ..................................... 474
Skoda's theorem ............................................... 475
Lecture 4 Asymptotic constructions ........................... 479
Asymptotic multiplier ideals .................................. 479
Variants ...................................................... 482
Étale multiplicativity of plurigenera ......................... 484
A comparison theorem for symbolic powers ...................... 485
Lecture 5 Extension theorems and deformation invariance of
plurigenera ........................................ 487
Bibliography .................................................. 493
János Kollár
Exercises in the Birational Geometry of Algebraic Varieties ... 495
1 Birational classification of algebraic surfaces ............ 497
2 Naive minimal models ....................................... 498
3 The cone of curves ......................................... 503
4 Singularities .............................................. 508
5 Flips ...................................................... 513
6 Minimal models ............................................. 518
Bibliography ............................................... 523
Christopher D. Hacon
Higher Dimensional Minimal Model Program for Varieties of
Log General Type .............................................. 525
Introduction .................................................. 527
Lecture 1 Pl-flips ........................................... 531
Lecture 2 Multiplier ideal sheaves ........................... 535
Asymptotic multiplier ideal sheaves ........................... 538
Extending pluricanonical forms ................................ 540
Lecture 3 Finite generation of the restricted algebra ........ 545
Rationality of the restricted algebra ......................... 545
Proof of (1.10) ............................................... 546
Lecture 4 The minimal model program with scaling ............. 547
Solutions to the exercises .................................... 551
Bibliography .................................................. 555
Alessio Corti, Paul Hacking, János Kollár, Robert
Lazarsfeld, and Mircea Mustaţă
Lectures on Flips and Minimal Models .......................... 557
Lecture 1 Extension theorems ................................. 561
1.1 Multiplier and adjoint ideals ............................ 561
1.2 Proof of the Main Lemma .................................. 563
Lecture 2 Existence of flips I ............................... 565
2.1 The setup ................................................ 565
2.2 Adjoint algebras ......................................... 566
2.3 The Hacon-McKernan extension theorem ..................... 567
2.4 The restricted algebra as an adjoint algebra ............. 567
Lecture 3 Existence of flips II .............................. 571
Lecture 4 Notes on Birkar-Cascini-Hacon-McKernan ............. 575
4.1 Comparison of 3 MMP's .................................... 576
4.2 MMP with scaling ......................................... 578
4.3 MMP with scaling near |Δ| ................................ 578
4.4 Bending it like BCHM ..................................... 579
4.5 Finiteness of models ..................................... 581
Bibliography ............................................. 583
|