Preface
John Hamad ...................................................... V
References ..................................................... IX
Part I Random Matrices, Random Processes and Integrable Models
1 Random and Integrable Models in Mathematics and Physics
Pierre van Moerbeke .......................................... 3
1.1 Permutations, Words, Generalized Permutations and
Percolation ............................................. 4
1.1.1 Longest Increasing Subsequences in
Permutations, Words and Generalized
Permutations ..................................... 4
1.1.2 Young Diagrams and Schur Polynomials ............. 6
1.1.3 Robinson-Schensted-Knuth Correspondence for
Generalized Permutations ......................... 9
1.1.4 The Cauchy Identity ............................. 11
1.1.5 Uniform Probability on Permutations,
Plancherel Measure and Random Walks ............. 13
1.1.6 Probability Measure on Words .................... 22
1.1.7 Generalized Permutations, Percolation and
Growth Models ................................... 24
1.2 Probability on Partitions, Toeplitz and Fredholm
Determinants ........................................... 33
1.2.1 Probability on Partitions Expressed as
Toeplitz Determinants ........................... 35
1.2.2 The Calculus of Infinite Wedge Spaces ........... 39
1.2.3 Probability on Partitions Expressed as
Fredholm Determinants ........................... 44
1.2.4 Probability on Partitions Expressed as U(n)
Integrals ....................................... 48
1.3 Examples ............................................... 50
1.3.1 Plancherel Measure and Gessel's Theorem ......... 50
1.3.2 Probability on Random Words ..................... 54
1.3.3 Percolation ..................................... 56
1.4 Limit Theorems ......................................... 60
1.4.1 Limit for Plancherel Measure .................... 60
1.4.2 Limit Theorem for Longest Increasing Sequences .. 64
1.4.3 Limit Theorem for the Geometrically
Distributed Percolation Model, when One Side
of the Matrix Tends to ∞ ........................ 67
1.4.4 Limit Theorem for the Geometrically Distributed
Percolation Model, when Both Sides of the
Matrix Tend to ∞ ................................ 71
1.4.5 Limit Theorem for the Exponentially Distributed
Percolation Model, when Both Sides of the
Matrix tend to ∞ ................................ 75
1.5 Orthogonal Polynomials for a Time-Dependent Weight
and the KP Equation .................................... 76
1.5.1 Orthogonal Polynomials .......................... 76
1.5.2 Time-Dependent Orthogonal Polynomials and the
KP Equation ..................................... 81
1.6 Virasoro Constraints ................................... 88
1.6.1 Virasoro Constraints for β-Integrals ........... 88
1.6.2 Examples ........................................ 93
1.7 Random Matrices ........................................ 96
1.7.1 Haar Measure on the Space 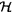 n of Hermitian
Matrices ........................................ 96
1.7.2 Random Hermitian Ensemble ....................... 99
1.7.3 Reproducing Kernels ............................ 102
1.7.4 Correlations and Fredholm Determinants ......... 104
1.8 The Distribution of Hermitian Matrix Ensembles ........ 108
1.8.1 Classical Hermitian Matrix Ensembles ........... 108
1.8.2 The Probability for the Classical Hermitian
Random Ensembles and PDEs Generalizing
Painleve ....................................... 113
1.8.3 Chazy and Painleve Equations ................... 119
1.9 Large Hermitian Matrix Ensembles ...................... 120
1.9.1 Equilibrium Measure for GUE and Wigner's
Semi-Circle .................................... 120
1.9.2 Soft Edge Scaling Limit for GUE and the
Tracy-Widom Distribution ....................... 122
References ............................................ 128
2 Integrable Systems, Random Matrices, and Random Processes
Mark Adler ................................................. 131
2.1 Matrix Integrals and Solitons ......................... 134
2.1.1 Random Matrix Ensembles ........................ 134
2.1.2 Large H-limits ................................. 137
2.1.3 KP Hierarchy ................................... 139
2.1.4 Vertex Operators, Soliton Formulas and
Fredholm Determinants .......................... 141
2.1.5 Virasoro Relations Satisfied by the Fredholm
Determinant .................................... 144
2.1.6 Differential Equations for the Probability in
Scaling Limits ................................. 146
2.2 Recursion Relations for Unitary Integrals ............. 151
2.2.1 Results Concerning Unitary Integrals ........... 151
2.2.2 Examples from Combinatorics .................... 154
2.2.3 Bi-orthogonal Polynomials on the Circle and
the Toeplitz Lattice ........................... 157
2.2.4 Virasoro Constraints and Difference Relations .. 159
2.2.5 Singularity Confinement of Recursion
Relations ...................................... 163
2.3 Coupled Random Matrices and the 2-Toda Lattice ........ 167
2.3.1 Main Results for Coupled Random Matrices ....... 167
2.3.2 Link with the 2-Toda Hierarchy ................. 168
2.3.3 L-U Decomposition of the Moment Matrix,
Bi-orthogonal Polynomials and 2-Toda Wave
Operators ...................................... 171
2.3.4 Bilinear Identities and τ-function PDEs ........ 174
2.3.5 Virasoro Constraints for the τ-functions ....... 176
2.3.6 Consequences of the Virasoro Relations ......... 179
2.3.7 Final Equations ................................ 181
2.4 Dyson Brownian Motion and the Airy Process ............ 182
2.4.1 Processes ...................................... 182
2.4.2 PDEs and Asymptotics for the Processes ......... 189
2.4.3 Proof of the Results ........................... 192
2.5 The Pearcey Distribution .............................. 199
2.5.1 GUE with an External Source and Brownian
Motion ......................................... 199
2.5.2 MOPS and a Riemann-Hilbert Problem ............. 202
2.5.3 Results Concerning Universal Behavior .......... 204
2.5.4 3-KP Deformation of the Random Matrix
Problem ........................................ 208
2.5.5 Virasoro Constraints for the Integrable
Deformations ................................... 213
2.5.6 A PDE for the Gaussian Ensemble with External
Source and the Pearcey PDE ..................... 218
A Hirota Symbol Residue Identity ...................... 221
References ................................................. 223
Part II Random Matrices and Applications
3 Integral Operators in Random Matrix Theory
Harold Widom ............................................... 229
3.1 Hilbert-Schmidt and Trace Class Operators. Trace and
Determinant. Fredholm Determinants of Integral
Operators ............................................. 229
3.2 Correlation Functions and Kernels of Integral
Operators. Spacing Distributions as Operator
Determinants. The Sine and Airy Kernels ............... 238
3.3 Differential Equations for Distribution Functions
Arising in Random Matrix Theory. Representations in
Terms of Painlevé Functions ........................... 243
References ................................................. 249
4 Lectures on Random Matrix Models
Pavel M. Bleher ............................................ 251
4.1 Random Matrix Models and Orthogonal Polynomials ....... 252
4.1.1 Unitary Ensembles of Random Matrices ........... 252
4.1.2 The Riemann-Hilbert Problem for Orthogonal
Polynomials .................................... 260
4.1.3 Distribution of Eigenvalues and Equilibrium
Measure ........................................ 263
4.2 Large N Asymptotics of Orthogonal Polynomials.
The Riemann-Hilbert Approach .......................... 267
4.2.1 Heine's Formula for Orthogonal Polynomials ..... 267
4.2.2 First Transformation of the RH Problem ......... 269
4.2.3 Second Transformation of the RHP: Opening of
Lenses ......................................... 271
4.2.4 Model RHP ...................................... 272
4.2.5 Construction of a Parametrix at Edge Points .... 280
4.2.6 Third and Final Transformation of the RHP ...... 286
4.2.7 Solution of the RHP for RN(z) .................. 287
4.2.8 Asymptotics of the Recurrent Coefficients ...... 288
4.2.9 Universality in the Random Matrix Model ........ 291
4.3 Double Scaling Limit in a Random Matrix Model ......... 294
4.3.1 Ansatz of the Double Scaling Limit ............. 294
4.3.2 Construction of the Parametrix in ΩWKB ......... 297
4.3.3 Construction of the Parametrix near the
Turning Points ................................. 299
4.3.4 Construction of the Parametrix near the
Critical Point ................................. 300
4.4 Large N Asymptotics of the Partition Function of
Random
Matrix Models ......................................... 308
4.4.1 Partition Function ............................. 308
4.4.2 Analyticity of the Free Energy for Regular V ... 310
4.4.3 Topological Expansion .......................... 311
4.4.4 One-Sided Analyticity at a Critical Point ...... 313
4.4.5 Double Scaling Limit of the Free Energy ........ 315
4.5 Random Matrix Model with External Source .............. 315
4.5.1 Random Matrix Model with External Source and
Multiple Orthogonal Polynomials ................ 315
4.5.2 Gaussian Matrix Model with External Source
and Non-Intersecting Brownian Bridges .......... 321
4.5.3 Gaus an Model with External Source. Main
Results ........................................ 322
4.5.4 Construction of a Parametrix in the Case
α > 1 .......................................... 326
4.5.5 Construction of a Parametrix in the Case
α < 1 .......................................... 333
4.5.6 Double Scaling Limit at a = 1 .................. 340
4.5.7 Concluding Remarks ............................. 346
References ........................................... 347
5 Large N Asymptotics in Random Matrices
Alexander R. Its ........................................... 351
5.1 The RH Representation of the Orthogonal Polynomials
and Matrix Models ..................................... 351
5.1.1 Introduction ................................... 351
5.1.2 The RH Representation of the Orthogonal
Polynomials .................................... 355
5.1.3 Elements of the RH Theory ...................... 360
5.2 The Asymptotic Analysis of the RH Problem. The
DKMVZ Method .......................................... 373
5.2.1 A Naive Approach ............................... 373
5.2.2 The g-Function ................................. 373
5.2.3 Construction of the g-Function ................. 378
5.3 The Parametrix at the End Points. The Conclusion of
the Asymptotic Analysis ............................... 383
5.3.1 The Model Problem Near z = z0 .................. 383
5.3.2 Solution of the Model Problem .................. 386
5.3.3 The Final Formula for the Parametrix ........... 390
5.3.4 The Conclusion of the Asymptotic Analysis ...... 391
5.4 The Critical Case. The Double Scaling Limit and the
Second Painleve Equation .............................. 394
5.4.1 The Parametrix at z = 0 ........................ 394
5.4.2 The Conclusion of the Asymptotic Analysis
in the Critical Case ........................... 399
5.4.3 Analysis of the RH Problem (1C)-(3C). The
Second Painleve Equation ....................... 403
5.4.4 The Painleve Asymptotics of the Recurrence
Coefficients ................................... 406
References ................................................. 412
6 Formal Matrix Integrals and Combinatorics of Maps
B. Eynard .................................................. 415
6.1 Introduction .......................................... 415
6.2 Formal Matrix Integrals ............................... 417
6.2.1 Combinatorics of Maps .......................... 419
6.2.2 Topological Expansion .......................... 423
6.3 Loop Equations ........................................ 423
6.4 Examples .............................................. 429
6.4.1 1-Matrix Model ................................. 429
6.4.2 2-Matrix Model ................................. 431
6.4.3 Chain of Matrices .............................. 434
6.4.4 Closed Chain of Matrices ....................... 435
6.4.5 O(n) Model ..................................... 435
6.4.6 Potts Model .................................... 437
6.4.7 3-Color Model .................................. 438
6.4.8 6-Vertex Model ................................. 438
6.4.9 ADE Models ..................................... 438
6.4.10 ABAB Models .................................... 439
6.5 Discussion ............................................ 439
6.5.1 Summary of Some Known Results .................. 439
6.5.2 Some Open Problems ............................. 440
References ................................................. 441
7 Application of Random Matrix Theory to Multivariate
Statistics
Momar Dieng and Craig A. Tracy ............................. 443
7.1 Multivariate Statistics ............................... 443
7.1.1 Wishart Distribution ........................... 443
7.1.2 An Example with ∑ ≠ cIp ........................ 446
7.2 Edge Distribution Functions ........................... 448
7.2.1 Summary of Fredholm Determinant
Representations ................................ 448
7.2.2 Universality Theorems .......................... 449
7.3 Painleve Representations: A Summary ................... 451
7.4 Preliminaries ......................................... 454
7.4.1 Determinant Matters ............................ 454
7.4.2 Recursion Formula for the Eigenvalue
Distributions .................................. 455
7.5 The Distribution of the mth Largest Eigenvalue in
the GUE ............................................... 458
7.5.1 The Distribution Function as a Fredholm
Determinant .................................... 458
7.5.2 Edge Scaling and Differential Equations ........ 459
7.6 The Distribution of the mth Largest Eigenvalue in
the GSE ............................................... 463
7.6.1 The Distribution Function as a Fredholm
Determinant .................................... 463
7.6.2 Gaussian Specialization ........................ 468
7.6.3 Edge Scaling ................................... 474
7.7 The Distribution of the mth Largest Eigenvalue in
the GOE ............................................... 481
7.7.1 The Distribution Function as a Fredholm
Determinant .................................... 481
7.7.2 Gaussian Specialization ........................ 486
7.7.3 Edge Scaling ................................... 490
7.8 An Interlacing Property ............................... 499
7.9 Numerics .............................................. 503
7.9.1 Partial Derivatives of q(x, λ) ................. 503
7.9.2 Algorithms ..................................... 503
7.9.3 Tables ......................................... 504
References ................................................. 505
Index ......................................................... 509 n of Hermitian
Matrices ........................................ 96
1.7.2 Random Hermitian Ensemble ....................... 99
1.7.3 Reproducing Kernels ............................ 102
1.7.4 Correlations and Fredholm Determinants ......... 104
1.8 The Distribution of Hermitian Matrix Ensembles ........ 108
1.8.1 Classical Hermitian Matrix Ensembles ........... 108
1.8.2 The Probability for the Classical Hermitian
Random Ensembles and PDEs Generalizing
Painleve ....................................... 113
1.8.3 Chazy and Painleve Equations ................... 119
1.9 Large Hermitian Matrix Ensembles ...................... 120
1.9.1 Equilibrium Measure for GUE and Wigner's
Semi-Circle .................................... 120
1.9.2 Soft Edge Scaling Limit for GUE and the
Tracy-Widom Distribution ....................... 122
References ............................................ 128
2 Integrable Systems, Random Matrices, and Random Processes
Mark Adler ................................................. 131
2.1 Matrix Integrals and Solitons ......................... 134
2.1.1 Random Matrix Ensembles ........................ 134
2.1.2 Large H-limits ................................. 137
2.1.3 KP Hierarchy ................................... 139
2.1.4 Vertex Operators, Soliton Formulas and
Fredholm Determinants .......................... 141
2.1.5 Virasoro Relations Satisfied by the Fredholm
Determinant .................................... 144
2.1.6 Differential Equations for the Probability in
Scaling Limits ................................. 146
2.2 Recursion Relations for Unitary Integrals ............. 151
2.2.1 Results Concerning Unitary Integrals ........... 151
2.2.2 Examples from Combinatorics .................... 154
2.2.3 Bi-orthogonal Polynomials on the Circle and
the Toeplitz Lattice ........................... 157
2.2.4 Virasoro Constraints and Difference Relations .. 159
2.2.5 Singularity Confinement of Recursion
Relations ...................................... 163
2.3 Coupled Random Matrices and the 2-Toda Lattice ........ 167
2.3.1 Main Results for Coupled Random Matrices ....... 167
2.3.2 Link with the 2-Toda Hierarchy ................. 168
2.3.3 L-U Decomposition of the Moment Matrix,
Bi-orthogonal Polynomials and 2-Toda Wave
Operators ...................................... 171
2.3.4 Bilinear Identities and τ-function PDEs ........ 174
2.3.5 Virasoro Constraints for the τ-functions ....... 176
2.3.6 Consequences of the Virasoro Relations ......... 179
2.3.7 Final Equations ................................ 181
2.4 Dyson Brownian Motion and the Airy Process ............ 182
2.4.1 Processes ...................................... 182
2.4.2 PDEs and Asymptotics for the Processes ......... 189
2.4.3 Proof of the Results ........................... 192
2.5 The Pearcey Distribution .............................. 199
2.5.1 GUE with an External Source and Brownian
Motion ......................................... 199
2.5.2 MOPS and a Riemann-Hilbert Problem ............. 202
2.5.3 Results Concerning Universal Behavior .......... 204
2.5.4 3-KP Deformation of the Random Matrix
Problem ........................................ 208
2.5.5 Virasoro Constraints for the Integrable
Deformations ................................... 213
2.5.6 A PDE for the Gaussian Ensemble with External
Source and the Pearcey PDE ..................... 218
A Hirota Symbol Residue Identity ...................... 221
References ................................................. 223
Part II Random Matrices and Applications
3 Integral Operators in Random Matrix Theory
Harold Widom ............................................... 229
3.1 Hilbert-Schmidt and Trace Class Operators. Trace and
Determinant. Fredholm Determinants of Integral
Operators ............................................. 229
3.2 Correlation Functions and Kernels of Integral
Operators. Spacing Distributions as Operator
Determinants. The Sine and Airy Kernels ............... 238
3.3 Differential Equations for Distribution Functions
Arising in Random Matrix Theory. Representations in
Terms of Painlevé Functions ........................... 243
References ................................................. 249
4 Lectures on Random Matrix Models
Pavel M. Bleher ............................................ 251
4.1 Random Matrix Models and Orthogonal Polynomials ....... 252
4.1.1 Unitary Ensembles of Random Matrices ........... 252
4.1.2 The Riemann-Hilbert Problem for Orthogonal
Polynomials .................................... 260
4.1.3 Distribution of Eigenvalues and Equilibrium
Measure ........................................ 263
4.2 Large N Asymptotics of Orthogonal Polynomials.
The Riemann-Hilbert Approach .......................... 267
4.2.1 Heine's Formula for Orthogonal Polynomials ..... 267
4.2.2 First Transformation of the RH Problem ......... 269
4.2.3 Second Transformation of the RHP: Opening of
Lenses ......................................... 271
4.2.4 Model RHP ...................................... 272
4.2.5 Construction of a Parametrix at Edge Points .... 280
4.2.6 Third and Final Transformation of the RHP ...... 286
4.2.7 Solution of the RHP for RN(z) .................. 287
4.2.8 Asymptotics of the Recurrent Coefficients ...... 288
4.2.9 Universality in the Random Matrix Model ........ 291
4.3 Double Scaling Limit in a Random Matrix Model ......... 294
4.3.1 Ansatz of the Double Scaling Limit ............. 294
4.3.2 Construction of the Parametrix in ΩWKB ......... 297
4.3.3 Construction of the Parametrix near the
Turning Points ................................. 299
4.3.4 Construction of the Parametrix near the
Critical Point ................................. 300
4.4 Large N Asymptotics of the Partition Function of
Random
Matrix Models ......................................... 308
4.4.1 Partition Function ............................. 308
4.4.2 Analyticity of the Free Energy for Regular V ... 310
4.4.3 Topological Expansion .......................... 311
4.4.4 One-Sided Analyticity at a Critical Point ...... 313
4.4.5 Double Scaling Limit of the Free Energy ........ 315
4.5 Random Matrix Model with External Source .............. 315
4.5.1 Random Matrix Model with External Source and
Multiple Orthogonal Polynomials ................ 315
4.5.2 Gaussian Matrix Model with External Source
and Non-Intersecting Brownian Bridges .......... 321
4.5.3 Gaus an Model with External Source. Main
Results ........................................ 322
4.5.4 Construction of a Parametrix in the Case
α > 1 .......................................... 326
4.5.5 Construction of a Parametrix in the Case
α < 1 .......................................... 333
4.5.6 Double Scaling Limit at a = 1 .................. 340
4.5.7 Concluding Remarks ............................. 346
References ........................................... 347
5 Large N Asymptotics in Random Matrices
Alexander R. Its ........................................... 351
5.1 The RH Representation of the Orthogonal Polynomials
and Matrix Models ..................................... 351
5.1.1 Introduction ................................... 351
5.1.2 The RH Representation of the Orthogonal
Polynomials .................................... 355
5.1.3 Elements of the RH Theory ...................... 360
5.2 The Asymptotic Analysis of the RH Problem. The
DKMVZ Method .......................................... 373
5.2.1 A Naive Approach ............................... 373
5.2.2 The g-Function ................................. 373
5.2.3 Construction of the g-Function ................. 378
5.3 The Parametrix at the End Points. The Conclusion of
the Asymptotic Analysis ............................... 383
5.3.1 The Model Problem Near z = z0 .................. 383
5.3.2 Solution of the Model Problem .................. 386
5.3.3 The Final Formula for the Parametrix ........... 390
5.3.4 The Conclusion of the Asymptotic Analysis ...... 391
5.4 The Critical Case. The Double Scaling Limit and the
Second Painleve Equation .............................. 394
5.4.1 The Parametrix at z = 0 ........................ 394
5.4.2 The Conclusion of the Asymptotic Analysis
in the Critical Case ........................... 399
5.4.3 Analysis of the RH Problem (1C)-(3C). The
Second Painleve Equation ....................... 403
5.4.4 The Painleve Asymptotics of the Recurrence
Coefficients ................................... 406
References ................................................. 412
6 Formal Matrix Integrals and Combinatorics of Maps
B. Eynard .................................................. 415
6.1 Introduction .......................................... 415
6.2 Formal Matrix Integrals ............................... 417
6.2.1 Combinatorics of Maps .......................... 419
6.2.2 Topological Expansion .......................... 423
6.3 Loop Equations ........................................ 423
6.4 Examples .............................................. 429
6.4.1 1-Matrix Model ................................. 429
6.4.2 2-Matrix Model ................................. 431
6.4.3 Chain of Matrices .............................. 434
6.4.4 Closed Chain of Matrices ....................... 435
6.4.5 O(n) Model ..................................... 435
6.4.6 Potts Model .................................... 437
6.4.7 3-Color Model .................................. 438
6.4.8 6-Vertex Model ................................. 438
6.4.9 ADE Models ..................................... 438
6.4.10 ABAB Models .................................... 439
6.5 Discussion ............................................ 439
6.5.1 Summary of Some Known Results .................. 439
6.5.2 Some Open Problems ............................. 440
References ................................................. 441
7 Application of Random Matrix Theory to Multivariate
Statistics
Momar Dieng and Craig A. Tracy ............................. 443
7.1 Multivariate Statistics ............................... 443
7.1.1 Wishart Distribution ........................... 443
7.1.2 An Example with ∑ ≠ cIp ........................ 446
7.2 Edge Distribution Functions ........................... 448
7.2.1 Summary of Fredholm Determinant
Representations ................................ 448
7.2.2 Universality Theorems .......................... 449
7.3 Painleve Representations: A Summary ................... 451
7.4 Preliminaries ......................................... 454
7.4.1 Determinant Matters ............................ 454
7.4.2 Recursion Formula for the Eigenvalue
Distributions .................................. 455
7.5 The Distribution of the mth Largest Eigenvalue in
the GUE ............................................... 458
7.5.1 The Distribution Function as a Fredholm
Determinant .................................... 458
7.5.2 Edge Scaling and Differential Equations ........ 459
7.6 The Distribution of the mth Largest Eigenvalue in
the GSE ............................................... 463
7.6.1 The Distribution Function as a Fredholm
Determinant .................................... 463
7.6.2 Gaussian Specialization ........................ 468
7.6.3 Edge Scaling ................................... 474
7.7 The Distribution of the mth Largest Eigenvalue in
the GOE ............................................... 481
7.7.1 The Distribution Function as a Fredholm
Determinant .................................... 481
7.7.2 Gaussian Specialization ........................ 486
7.7.3 Edge Scaling ................................... 490
7.8 An Interlacing Property ............................... 499
7.9 Numerics .............................................. 503
7.9.1 Partial Derivatives of q(x, λ) ................. 503
7.9.2 Algorithms ..................................... 503
7.9.3 Tables ......................................... 504
References ................................................. 505
Index ......................................................... 509
|